Question: Find the sum of the series ∑n=1∞12n12 correct to three decimal places.Step 1Consider that f(x)=12x12 is positive and continuous for x>0.To decide if f(x)=12x12 is also decreasing, we can examine the derivative f'(x)=-144x13,-144x13.Step 2Examining the derivative, we have f'(x)=-144x-13=-144x13.Since the denominator is always positive on (0,∞) then
Find the sum the series correct three decimal places.StepConsider that positive and continuous fordecide also decreasing, can examine the derivativeStepExamining the derivative, haveSince the denominator always positive then always negative negative.StepSince always negative, then decreasing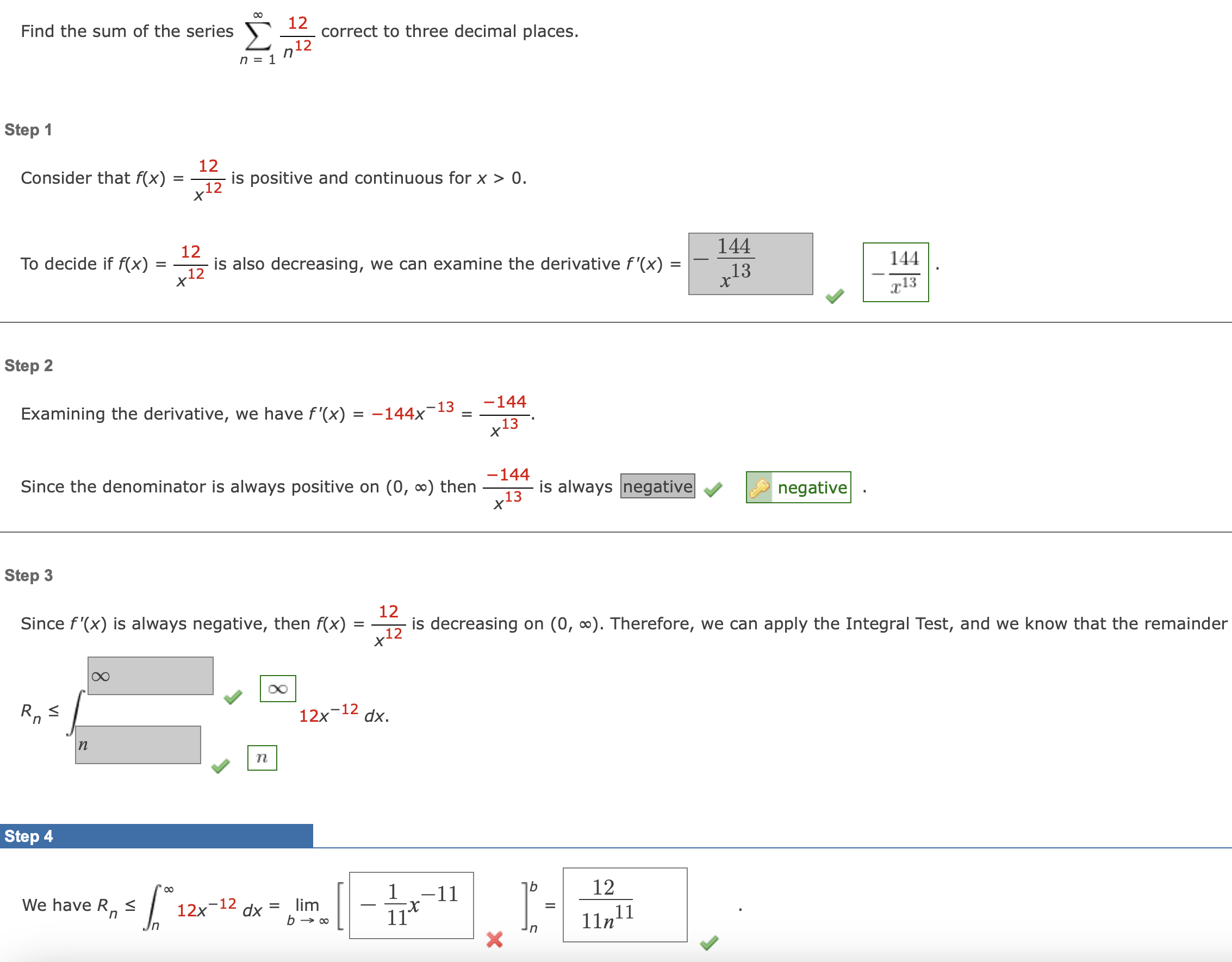
- This question hasn't been solved yet!Not what you’re looking for?Submit your question to a subject-matter expert.
Not the question you’re looking for?
Post any question and get expert help quickly.